User:Lieven Van Speybroeck/Prototyping/7-Turtle Mondriaan: Difference between revisions
(Created page with "File:Bob_Mondriaan1.png File:Bob_Mondriaan2.png") |
No edit summary |
||
| (16 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
Presenting Bob Mondriaan!<br> | |||
The idea is that the same code generates different 'paintings'. I tried using Inkscape to get colors involved, but somehow, things are not working properly.<br> | |||
So for now, I can show the TurtleWorld output (colors are quite crucial, I know): | |||
[[File:Bob_Mondriaan1.png]] | [[File:Bob_Mondriaan1.png]] | ||
[[File:Bob_Mondriaan3.png]] | |||
[[File:Bob_Mondriaan2.png]] | [[File:Bob_Mondriaan2.png]] | ||
These images consist of full rectangles that could be filled with a random color (obviously white, blue, red or yellow). | |||
The code is a bit of a mess, and I'm still thinking of how I could 'automate' more instead of all the hardcoding: | |||
<source lang="python"> | |||
from TurtleWorld import * | |||
import math | |||
import random | |||
world = TurtleWorld() | |||
bob = Turtle() | |||
bob.delay = 0.01 | |||
print bob | |||
def rectangle(t, width, height): | |||
for i in range(2): | |||
fd(t, width) | |||
rt(t) | |||
fd(t, height) | |||
rt(t) | |||
def mondriaan(t, scale): | |||
# omtrek | |||
rectangle(t, scale, scale) | |||
newWidth = random.randint(3,scale) | |||
newHeight = random.randint(3,scale) | |||
# rectangle 1 | |||
rectangle(t, newWidth, newHeight) | |||
fd(t, newWidth) | |||
restWidth = scale - newWidth | |||
restHeight = scale - newHeight | |||
# rectangle 2 | |||
rectangle(t, restWidth, newHeight/2) | |||
rt(t) | |||
fd(t, newHeight/2) | |||
lt(t) | |||
# rectangle 3 | |||
for i in range(2): | |||
rectangle(t, restWidth/2, newHeight/2) | |||
fd(t, restWidth/2) | |||
rt(t) | |||
fd(t, newHeight/2) | |||
#rectangle 4 | |||
rectangle(t, restHeight/2.5, restWidth) | |||
rt(t) | |||
fd(t, restWidth) | |||
lt(t) | |||
#rectangle 5 | |||
for i in range(2): | |||
rectangle(t, restHeight/2.5, newWidth/2) | |||
fd(t, restHeight/2.5) | |||
rt(t) | |||
fd(t, newWidth/2) | |||
#rectangle 6 | |||
rectangle(t, newWidth/2, (restHeight/2.5)*2) | |||
fd(t, newWidth/2) | |||
lt(t) | |||
lt(t) | |||
#rectangle 7 | |||
rectangle(t, newWidth, (restHeight/2.5)*0.5) | |||
pu(t) | |||
fd(t, scale) | |||
rt(t) | |||
fd(t, (restHeight/2.5)*0.5) | |||
rt(t) | |||
pd(t) | |||
#rectangle 8 | |||
rectangle(t, restWidth, (restHeight/2.5)*1.5) | |||
mondriaan(bob, 400) | |||
wait_for_user() | |||
</source> | |||
SVG code (not working... but we could have a look at it) | |||
<source lang="python"> | |||
import random | |||
import math | |||
def rectangle(width, height): | |||
for i in range(2): | |||
pd() | |||
fd(width) | |||
rt(90) | |||
fd(height) | |||
rt(90) | |||
colors = ['255,0.0', '0,255,0', '0,0,255', '255,255,255', '0,0,0'] | |||
color = choice(color) | |||
styles['fill'] = color | |||
(pu) | |||
def mondriaan(scale): | |||
# omtrek | |||
rectangle(scale, scale) | |||
newWidth = random.randint(3,scale) | |||
newHeight = random.randint(3,scale) | |||
# rechthoek 1 | |||
rectangle(newWidth, newHeight) | |||
fd(newWidth) | |||
restWidth = scale - newWidth | |||
restHeight = scale - newHeight | |||
# rechthoek 2 | |||
rectangle(restWidth, newHeight/2) | |||
rt(90) | |||
fd(newHeight/2) | |||
lt(90) | |||
# rechthoek 3 | |||
for i in range(2): | |||
rectangle(restWidth/2, newHeight/2) | |||
fd(restWidth/2) | |||
rt(90) | |||
fd(newHeight/2) | |||
#rechthoek 4 | |||
rectangle(restHeight/2.5, restWidth) | |||
rt(90) | |||
fd(restWidth) | |||
lt(90) | |||
#rechthoek 5 | |||
for i in range(2): | |||
rectangle(restHeight/2.5, newWidth/2) | |||
fd(restHeight/2.5) | |||
rt(90) | |||
fd(newWidth/2) | |||
#rechthoek 6 | |||
rectangle(newWidth/2, (restHeight/2.5)*2) | |||
fd(newWidth/2) | |||
lt(90) | |||
lt(90) | |||
#rechthoek 7 | |||
rectangle(newWidth, (restHeight/2.5)*0.5) | |||
pu() | |||
fd(scale) | |||
rt(90) | |||
fd((restHeight/2.5)*0.5) | |||
rt(90) | |||
pd() | |||
#rechthoek 8 | |||
rectangle(restWidth, (restHeight/2.5)*1.5) | |||
mondriaan(400) | |||
</source> | |||
Latest revision as of 11:06, 14 June 2011
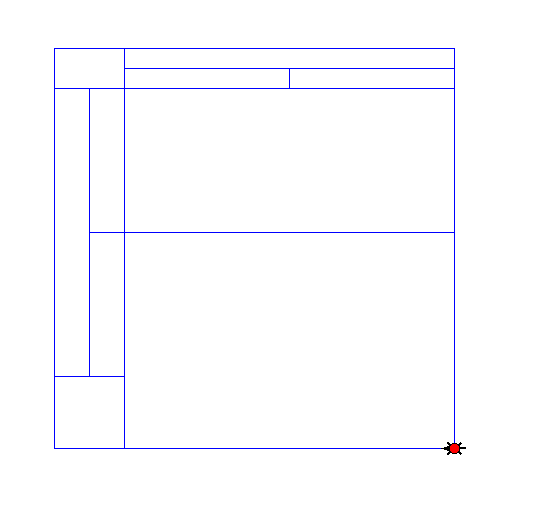
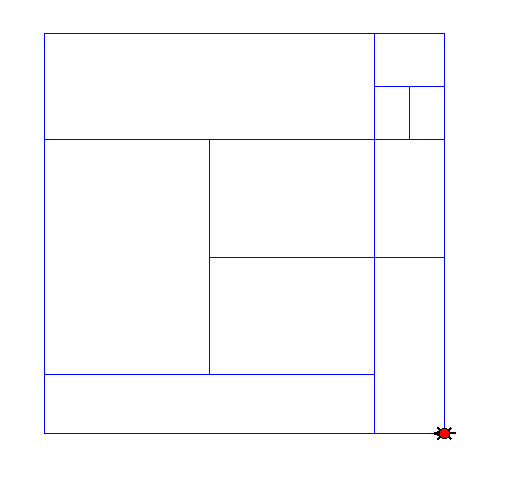
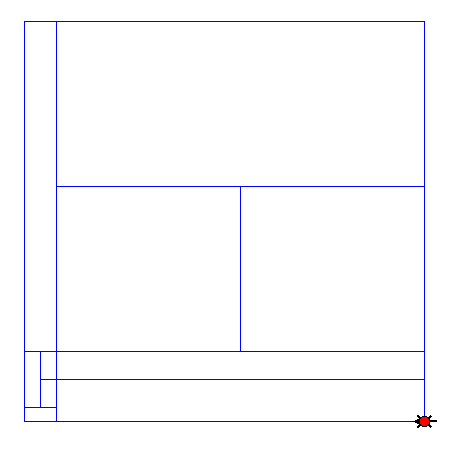
Presenting Bob Mondriaan!
The idea is that the same code generates different 'paintings'. I tried using Inkscape to get colors involved, but somehow, things are not working properly.
So for now, I can show the TurtleWorld output (colors are quite crucial, I know):
These images consist of full rectangles that could be filled with a random color (obviously white, blue, red or yellow).
The code is a bit of a mess, and I'm still thinking of how I could 'automate' more instead of all the hardcoding:
from TurtleWorld import *
import math
import random
world = TurtleWorld()
bob = Turtle()
bob.delay = 0.01
print bob
def rectangle(t, width, height):
for i in range(2):
fd(t, width)
rt(t)
fd(t, height)
rt(t)
def mondriaan(t, scale):
# omtrek
rectangle(t, scale, scale)
newWidth = random.randint(3,scale)
newHeight = random.randint(3,scale)
# rectangle 1
rectangle(t, newWidth, newHeight)
fd(t, newWidth)
restWidth = scale - newWidth
restHeight = scale - newHeight
# rectangle 2
rectangle(t, restWidth, newHeight/2)
rt(t)
fd(t, newHeight/2)
lt(t)
# rectangle 3
for i in range(2):
rectangle(t, restWidth/2, newHeight/2)
fd(t, restWidth/2)
rt(t)
fd(t, newHeight/2)
#rectangle 4
rectangle(t, restHeight/2.5, restWidth)
rt(t)
fd(t, restWidth)
lt(t)
#rectangle 5
for i in range(2):
rectangle(t, restHeight/2.5, newWidth/2)
fd(t, restHeight/2.5)
rt(t)
fd(t, newWidth/2)
#rectangle 6
rectangle(t, newWidth/2, (restHeight/2.5)*2)
fd(t, newWidth/2)
lt(t)
lt(t)
#rectangle 7
rectangle(t, newWidth, (restHeight/2.5)*0.5)
pu(t)
fd(t, scale)
rt(t)
fd(t, (restHeight/2.5)*0.5)
rt(t)
pd(t)
#rectangle 8
rectangle(t, restWidth, (restHeight/2.5)*1.5)
mondriaan(bob, 400)
wait_for_user()
SVG code (not working... but we could have a look at it)
import random
import math
def rectangle(width, height):
for i in range(2):
pd()
fd(width)
rt(90)
fd(height)
rt(90)
colors = ['255,0.0', '0,255,0', '0,0,255', '255,255,255', '0,0,0']
color = choice(color)
styles['fill'] = color
(pu)
def mondriaan(scale):
# omtrek
rectangle(scale, scale)
newWidth = random.randint(3,scale)
newHeight = random.randint(3,scale)
# rechthoek 1
rectangle(newWidth, newHeight)
fd(newWidth)
restWidth = scale - newWidth
restHeight = scale - newHeight
# rechthoek 2
rectangle(restWidth, newHeight/2)
rt(90)
fd(newHeight/2)
lt(90)
# rechthoek 3
for i in range(2):
rectangle(restWidth/2, newHeight/2)
fd(restWidth/2)
rt(90)
fd(newHeight/2)
#rechthoek 4
rectangle(restHeight/2.5, restWidth)
rt(90)
fd(restWidth)
lt(90)
#rechthoek 5
for i in range(2):
rectangle(restHeight/2.5, newWidth/2)
fd(restHeight/2.5)
rt(90)
fd(newWidth/2)
#rechthoek 6
rectangle(newWidth/2, (restHeight/2.5)*2)
fd(newWidth/2)
lt(90)
lt(90)
#rechthoek 7
rectangle(newWidth, (restHeight/2.5)*0.5)
pu()
fd(scale)
rt(90)
fd((restHeight/2.5)*0.5)
rt(90)
pd()
#rechthoek 8
rectangle(restWidth, (restHeight/2.5)*1.5)
mondriaan(400)